I am a Math Geek

It's true, I confess. On Sunday, we played Mexican Train with my aunt and uncle, which is a game of dominoes. Mexican Train uses a set of "double twelve" dominoes (I think that's the minimum; their set went up to 15). At home, I have a set of "double six" dominoes, but I remembered seeing in my set the hexagonal centerpiece similar to the one used in Mexican Train. So today I played a miniature game of Mexican Train with my mom, using what pieces we had available. It was a very short game. Curious, I felt compelled to calculate the mathematical formula that describes how many dominoes belong to a set.
If anyone cares (and I can't imagine who would), the number of dominoes in a set is the sum of the values of i from i=1 to i=n+1, where n represents the greatest number of spots in the set. This is represented by the formula:
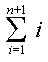
So a set that goes up to six spots contains 28 dominoes (1+2+3+4+5+6+7), and a set with twelve spots has 91 pieces.
If you're wondering why it's n+1 instead of just n, it's because dominoes have "blanks" (zeros).
grief weakens him.



